

Vol. 40 (Number 30) Year 2019. Page 26
DE LA TORRE-TORRES, Oscar V. 1; ÁLVAREZ-GARCÍA, José 2; SANTILLÁN-SALGADO, Roberto J. 3 & LÓPEZ HERRERA, Francisco 4
Received: 25/05/2019 • Approved: 03/09/2019 • Published 09/09/2019
4. Results: Empirical analysis
ABSTRACT: Nowadays, the average Mexican pension saver makes a noisy and uninformed investment decision of its Public pension fund (AFORE). This is due to AFORE’s marketing efforts or back-to-back activities. In the present paper, we propose the use of Markov-Switching models, in order to measure the AFORE performance in normal (crisis) or low (high) volatility time periods. With these measures, we simulated the correspondent fund selection. Our results show improvements in the long-term performance in the individuals’ pension savings. |
RESUMEN: Actualmente, el trabajador mexicano promedio hace una selección ruidosa y desinformada de su fondo pensiones (AFORE). Esto debido a esfuerzos mercadológicos o a actividades de tipo “back-to-back”. En el presente proponemos el empleo de modelos markovianos de cambio de régimen para medir el desempeño de las AFOREs en periodos normales (de crisis) o de baja (alta) volatilidad. Con estas mediciones, simulamos la correspondiente elección de fondo. Nuestros resultados muestran mejoras de desempeño en el largo plazo para los ahorradores. |
The Mexican government implemented important reforms to the country’s pensions system in 1997. Those reforms paved the way for a gradual migration from a conventional “defined benefit” (also called “pay-as-you-go”) system, then administered by a government entity, to a “defined contribution” system, in which private firms manage and invest the population’s retirement funds. The worker, the employer and the Federal Government make periodical contributions to the worker’s retirement account, and these resources are invested through specialized funds known as “Sociedades de Inversión Especializadas en Fondos para el Retiro” - Specialized Mutual Funds for Pensions - (SIEFOREs) in different types of securities, according to a regulatory framework.
Despite the favorable impact of this type reforms on domestic savings rates, on the deepening and diversification of domestic securities’ markets, and on healthier public finances, there is still much to be learned. For example, according to Patricia Peinado and Felipe Serrano (2011), there are important differences between the planned pension reforms, the actions implemented, and the effective results, depending on the criteria applied, including the eligibility conditions of future pensioners, defined retirement age, and others. It is clear that a better understanding of defined contribution pension systems is required.
This work studies the Mexican private pensions industry, and compares the historical performance of different SIEFOREs with the hypothetical performance of workers’ savings portfolios, had they had access to better SIEFOREs’ performance measures, information on prevailing market conditions, and had there not been legal constraints to the transfer of savings among SIEFOREs.
The experiment consists of the use of a two-regime Markov-Switching Sharpe Ratio (MS_SR) as information inputs available to a theoretical saver that makes optimal portfolio allocation decisions, depending on the prevailing market regime. These theoretical portfolios are then benchmarked with SIEFOREs’ historical performance during the period of analysis to highlight the cost of the existing limitations in savers’ available information, as well as the implicit cost of the rigidity imposed by the impediment to transfer savings from one SIEFORE to another more than once a year.
The following section presents some antecedents and reviews some relevant studies on defined contribution pension reforms in the world, with an emphasis on those of Latin American countries and the Mexican experience. The third section introduces the main methodological aspects of the study, including the construction of the Markov-Switching Sharpe Ratios, as well as an explanation of how that information may be used to make optimal investment decisions under different capital markets conditions. The fourth section reports the simulated performance results, assuming Markov-Switching Sharpe-Ratios are used to decide on the best possible allocation of investments among different SIEFOREs every month, and quantifies the cost of opportunity of more restricted information and regulatory constraints on the transfer of saving from one SIEFORE to another more than once a year. The final section presents some general conclusions, as well as guidelines for future research.
Progress in the medical sciences and the effects of technological progress on productivity have created the conditions for an aging population in many countries. The new demographic trends that characterize modern societies in the 21st century (a slowly growing and aging population) raise several relevant concerns for traditional defined benefit pension schemes and their long-term sustainability (Peinado and Serrano, 2014). New approaches are currently under consideration by social scientists and governments at large, and among different schemes, one that has received significant attention due to its consistency and adaptability to the new global demographic trends is the defined contribution pension system (John Williamson et al., 2012).
The first defined contribution pension system was introduced in Chile during the early 1980s as part of broader structural reforms to detonate economic growth and privatize that country’s extensive state-owned productive sector (Estelle James, 2005; Santillán-Salgado et al., 2010). The new Chilean pension system was called Administradoras de Fondos de Pensiones (AFP) –Pension Funds Managers–, and constituted an important component of Chile’s new economic model. During the following two decades, countries in Latin America, Eastern and Central Europe, and the Asian-Pacific region (Japan, Singapore, India) adopted similar systems (James 2005).
Defined contribution systems have also become increasingly popular in developed countries such as Canada, Ireland, the United Kingdom and the United States. Defined benefit plans have frequently been used in competitive labor markets as a way to retain highly skilled human capital. By contrast, defined contribution plans cannot fulfill the same task because workers’ account balances are fully portable, so workers have no incentive related to pension benefits to stay with their current employer. Notwithstanding, an increasing number of countries have migrated to defined contribution systems. For interested readers, John Turner and Gerard Hughes (2008) discuss the reason for the decline in defined benefit plans and the migration toward defined contribution plans in the more developed countries in much greater detail.
The work of Schmidt-Hebbel (1999) studies the way the substitution of a fully-funded pension system with a defined contribution pension system generates efficiency gains in different markets, contributes to higher savings, and results in increased economic growth. This work identifies the likely factor-market benefits of pension reform and its macroeconomic implications. The findings of this author are that defined benefit systems are consistently beneficial from a macro-economic perspective and suggest that Chile’s pension system had positive effects on the labor market, raised the country’s savings and improved factor productivity. He also quantifies the pension-fund system’s contribution to Chile’s economic growth rate and concludes that as much as one fourth of it may be explained by the reform.
In effect, strong evidence confirms the argument that defined contribution pension systems have supported the growth and increasing sophistication of financial markets in the countries that have adopted them. According to Hans Blommestein (1997), pension system reform in emerging markets positively influenced the development of a domestic institutional investors’ sector which, in turn, has supported the development of securities markets. This work compares the development of the institutional investors’ sector in emerging market economies with the experience of OECD members, identifies possible obstacles to the development of institutional investors and suggests policies to deal with these problems. Santillán-Salgado et al. (2010) study the role of the adoption of the AFP system in Chile during the early 1980s and quantify the relationship between the changes in the breadth and depth of the bond and stock markets and the growth of pension fund investments. They document that, not only did the size of the capital markets grow significantly during the decades that followed the adoption of the defined contributions system, but also the sophistication of the financial products and the number of intermediaries expanded considerably.
Beyond the macroeconomic benefits of defined contribution systems, an important concern of the studies on pension systems policy is to make sure the resources accumulated by future pensioners along their working life provide them with a satisfactory pension. However, the significant demographic differences and economic development conditions from one country to another make it almost impossible to generalize how to measure and project the performance of pension funds.
As the main interest of this work is centered on Mexico’s reformed pension system, the following paragraphs review previous research studies that have focused on SIEFORES in some detail. For instance, Adolfo Albo et al. (2007) develop demographic actuarial projections for the size and composition of the Mexican population, and relate them to the documented evolution of the economy through different periods, including economic crises and structural reform episodes, and conclude that the defined- contribution system has various opportunities for improvement, including the establishment of a national pension system that would overcome the fragmentation of different pension regimes in the country. These authors’ proposals are extensive and detailed and deserve careful attention by policy makers. However, one recommendation that requires urgent attention is the revision of the contribution that workers and employers should make to the system, currently at 8.1% of the worker’s income, below the average for Latin America of 8.7%.
In a similar vein, Javier Alonso et al. (2015) develop macroeconomic and actuarial projections to simulate the expected coverage and replacement rate of the SAR in Mexico for the period 2012-2050, based on demographic and economic forecasts, and report that the pension system limited improvements in coverage rates. In other words, the possibility of obtaining adequate pensions under the current system is restricted to those individuals who enjoy stable long-term employment and thus make significant contributions to their individual accounts every month. These authors also highlight the importance of financial education to improve the population’s savings decisions.
Calderón-Colín et al. (2009) extend the analysis and find that in “noisy” markets, such as Mexico’s defined benefits pension-fund industry, the number of participant competitors does not significantly reduce mark-ups, contrary to what would be expected in a competitive market. Their modeling exercise concludes, similar to Berstein, Solange and Ruiz (2005) that an increasing number of pension fund managers has not implied a fast mark-up reduction, raising important questions about the adequacy of the regulatory framework for the industry.
Eduardo Fuentes et al. (2010) review the late 1990s reforms to the Mexican pension system and discuss the fiscal benefits attributable to the new system. While they conclude that the various reforms implemented in 1997 clearly represent fiscal and economic benefits for the country, there is a need to increase the rate of replacement to make it converge with international standards. This last suggestion is intended to promote competition among pension-fund managers and to produce indirect benefits for pensioners. Lastly, they focus on the need to help low-income workers have more appropriate retirement pensions.
A representative sample of studies that deal with the financial performance of SIEFORES, and help contextualize the present work, include the those of Marissa Martínez and Francisco Venegas-Martínez (2014), who study the performance of Type 1 and Type 2 SIEFOREs with an equally weighted performance benchmark for each SIEFORE type and an ARIMA-GARCH model. They divide their study period into two sub periods: June 1997 - August 2004 and September 2004 - December 2010. Their results show that, in terms of mean-variance efficiency measured by Sharpe ratios, Type 2 SIEFORES underperform the most, compared with the conservative Type 1. This happens due to the high and asymmetric volatility of the time series. In their conclusions, they recommend, in line with this work’s proposal, to develop a better performance measure of SIEFOREs, and the need to inform pension-fund savers when market conditions are in a scenario of higher volatility and greater potential loss.
Óscar V. De la Torre et al. (2015) propose a minimum variance portfolio as a method to build a benchmark portfolio for defined contribution pension funds’ performance in Mexico. They perform three discrete event simulations with daily data from January 2002 to May 2013 and compare the results of the minimum variance portfolio with those of a Max Sharpe Ratio portfolio, and, lastly, with a linear combination of the minimum variance and Max Sharpe Ratio portfolios. Using Jeffery Bailey’s (1992) risk exposure, market representativeness, and turnover benchmark quality criteria, the authors conclude that the minimum variance portfolio is the preferred benchmark for publicly traded Mexican defined contribution pension funds.
Roberto J. Santillán-Salgado et al. (2016), along the line of work of Martínez-Preece and Venegas-Martínez (2014), study the performance of SIEFOREs in three different sub-periods (1997-2012, 2004-2012 and 2008-2012), and find fractional integration in their returns’ time-series, so they propose the use of ARMA-FIGARCH models to measure their long-term performance, as well as to model their volatility and returns.
These references confirm an increasing interest on the defined contribution pension systems structure and functioning. In the case of Mexico, several studies have focused on the performance of SIEFOREs, as the workers’ savings accumulation, including reinvested returns, will determine their retirement standard of living. We found no published evidence on the use of Markov-Switching Sharpe ratios in the mutual fund industry, specifically in the case of life-cycle mutual funds, 401K, or Latin American defined contribution pension funds, confirming the originality of the present proposal.
One of the most frequently used measures to evaluate a portfolio’s performance is the Sharpe Ratio, which is simply the risk premium (slope of the Securities Market Line) when the investment set includes a risk-free asset and the tangency portfolio of risky assets:

The approach followed in the empirical analysis is based on the generation of simulation results to support the argument that access to better quality information on the performance of SIEFOREs may give future pensioners the opportunity to improve their allocation decisions. Moreover, if, at the same time, institutional restrictions that limit the transference of savings among SIEFOREs to only once a year were eliminated, simulation results suggest the system could further improve its performance.
SIEFOREs are responsible for investing the pension savings of Mexican workers. There are five types of SIEFOREs: SB4 (or type 4 SIEFOREs), designed for savers aged under 36 years; SB3 (or type 3 SIEFOREs), that follow an investment policy appropriate for savers between 37 and 45 years; SB2 (or type 2 SIEFOREs), designed for a population with ages between 46 and 59 years; SB1 (or type 1 SIEFOREs), for individuals over 60; and SB0 (or type 0 SIEFOREs), for already retired individuals. The authorized investment policy for each SIEFORE type is presented in Table 1. This work’s analysis is centered on SB1 to SB4 type SIEFOREs, as SB0 type respond to a different portfolio management rationale since retired individuals are no longer interested in transferring their savings from one SIEFORE to another.
Table 1
Holding Limits by SIEFORE Type
(Current Regulation)
Asset type restrictions (min/max) |
SB0 |
SB1 |
SB2 |
SB3 |
SB4 |
Mexican Gov. bonds 1/ |
51%/100% |
51%/100% |
0%/100% |
0%/100% |
0%/100% |
Mexican corp. bonds 1/ |
0%/100% |
0%/100% |
0%/100% |
0%/100% |
0%/100% |
Mexican stocks |
0%/5% |
0%/5% |
0%/25% |
0%/30% |
0%/40% |
Gov. and corp. global bonds 2/ |
0%/100% |
0%/100% |
0%/100% |
0%/100% |
0%/100% |
Global equity markets 3/ |
0%/5% |
0%/5% |
0%/25% |
0%/30% |
0%/40% |
Commod. 4/ |
0% |
0% |
0%/5% |
0%/10% |
0%/10% |
FX risk limits |
|||||
Foreign Currency denominated securities |
0% |
0%/20% |
0%/20% |
0%/20% |
0%/20% |
Note: Percentages represent the minimum and maximum allowed in each category of investment vehicles.
Source: CONSAR (2016), https://www.gob.mx/consar.
Our sample of ten SIEFOREs includes those with historical data for the period from November 30, 2008, through December 30, 2014, a sampling decision intended to avoid the risk of a survivor bias in the analysis.
Table 2
List of SIEFORES
in the Sample
Azteca |
Inbursa |
Principal |
XXI Banorte |
Banamex |
Invercap |
Profuturo GNP |
|
Coppel |
Metlife |
SURA |
Source: CONSAR (2014).
The MS-SRs are calculated according to changes in volatility regimes, identified following Hamilton’s (1989) methodology. Sharpe ratios are used because they represent comprehensive performance measures, and because they express the expected returns premiums per unit of risk. Additionally, Maximum Sharpe Ratios (Max Sharpe Ratios) are estimated for each SIEFORE type and used as the benchmark against which individual SIEFOREs are compared. In other words, a Max Sharpe Ratio portfolio represents the optimal combination of assets that complies with the legal limits established for each category of financial assets, for each SIEFORE type. In that sense, the Max Sharpe Ratio portfolio is equivalent to the Tangency Portfolio, that is, the particular combination of risky financial assets that corresponds to the tangency point between the risky-assets’ efficient frontier, and the straight line that represents all possible combinations between the Risk-Free Asset and that particular portfolio. In other words, the Max Sharpe Ratio portfolio is an optimal portfolio for each SIEFORE type. The MS-SRs are used to identify which SIEFORE is more attractive within each given type, under different market regimes. To estimate the Max Sharpe Ratio benchmark portfolios, the investment-level restrictions established by CONSAR, according to Table 1, are operationalized using commercial public domain indices, as detailed in Table 3:
Table 3
Asset Type, Market Available
Securities, and Vendor
Asset type benchmark used herein |
Index |
Vendor |
Mexican Government bonds |
Valmer Government |
VALMER- Mexican Stock Exchange |
Mexican corporate bonds |
Valmer Corporate |
VALMER- Mexican Stock Exchange |
Mexican equity market |
IPC |
Mexican Stock Exchange |
Government and corporate global bonds |
World Bond Investment Grade ex MBS |
Citigroup Inc. |
Global equity markets |
MSCI World |
MSCI Inc. |
Commodities |
DJ-UBS commodity index |
Dow Jones – Citigroup Inc. |
Source: Authors’ own elaboration with publicly available data.
The model that determines the investment weights of the Max Sharpe Ratio portfolio solves the following mathematical problem:
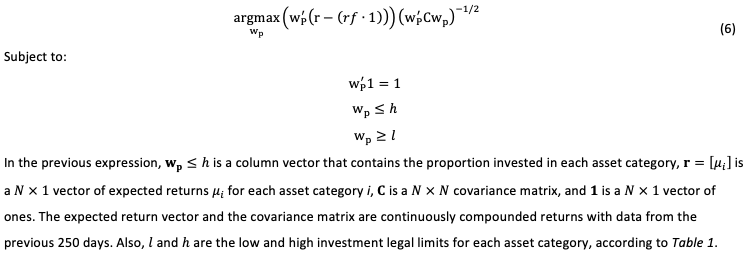
Table 4
Performance of Type 1 SIEFOREs During “Fair
Performance” and “Poor Performance” Periods
Panel A Gaussian MS Analysis for Type 1 SIEFOREs |
|||||
SIEFORE |
Net return (%) |
Expected return"fair perf." |
Expected return"poor perf." |
Expected risk "fair perf." |
Expected risk "poor perf." |
MaxS-SB1 |
72.8608 |
6.987*** |
20.641 |
2.1692 |
10.721 |
Azteca |
41.6939 |
6.2808*** |
1.8377 |
2.5344 |
8.1996 |
Banamex |
56.0163 |
7.9772*** |
2.1275 |
2.6799 |
8.885 |
Coppel |
37.9507 |
6.6128*** |
-0.3589 |
2.1561 |
7.1758 |
Inbursa |
42.5623 |
4.5389*** |
10.152*** |
0.4772 |
1.9336 |
Invercap |
49.9814 |
8.5699*** |
-9.2593 |
4.0682 |
17.688 |
Metlife |
46.2641 |
7.607*** |
-0.422 |
2.8267 |
9.2518 |
Principal |
49.2131 |
7.907*** |
0.3849 |
2.7015 |
8.2567 |
Profuturo GNP |
48.9634 |
8.6142*** |
-0.6648 |
2.8248 |
8.7931 |
SURA |
60.2435 |
7.786*** |
4.4733 |
2.9248 |
8.8978 |
XXI Banorte |
54.1112 |
7.7262*** |
1.8701 |
2.5587 |
8.4907 |
-----
Panel B an Example of the Ranking of Type 1 SIEFOREs on December 2014. |
|||||
SIEFORE ranking |
Sharpe ratio "fair perf." |
Sharpe ratio "poor perf. " |
Sharpe ranking "fair perf." |
Sharpe ranking "poor perf." |
Net return ranking |
MaxS-SB1 |
1.2216 |
1.4643 |
|||
Azteca |
0.7669 |
-0.3786 |
9 |
5 |
9 |
Banamex |
1.3583 |
-0.3168 |
2 |
3 |
2 |
Coppel |
1.0554 |
-0.7388 |
7 |
9 |
10 |
Inbursa |
0.4225 |
2.6942 |
10 |
1 |
8 |
Invercap |
1.0404 |
-0.8029 |
8 |
10 |
4 |
Metlife |
1.1568 |
-0.5798 |
6 |
7 |
7 |
Principal |
1.3214 |
-0.552 |
4 |
6 |
5 |
Profuturo GNP |
1.5141 |
-0.6377 |
1 |
8 |
6 |
SURA |
1.1792 |
-0.0527 |
5 |
2 |
1 |
XXI Banorte |
1.3245 |
-0.3618 |
3 |
4 |
3 |
* = Significant at 10%; ** = Significant at 5%; *** = Significant at 1%.
Note: Significance levels are reported for the Expected Return variables because the Quasi-Maximum Likelihood algorithm suggested in Hamilton (1994) was used in the estimations.
Source: Own elaboration with data retrieved from CONSAR (2014), https://www.gob.mx/ consar.
According to the net return ranking in Table 4, Coppel is the worst performer, and SURA the best. However, according to the MS-SR (Table 4), Profuturo-GNP (GNP hereafter), and Banamex are the best performers during “fair performance” periods (GNP is a “middle-rank performer” according to the net return method), but Inbursa and SURA are the best SIEFOREs during “poor performance” times. If savers were informed of which SIEFOREs are the best performers during “fair performance” and “poor performance” periods and had information about which regime prevails at every moment, they would be able to reallocate their pension savings and optimize the performance of their portfolio.
The MS-SRs and the Max Sharpe benchmark portfolio for each SIEFORE are obtained from the net returns calculated from the SIEFORE’s market prices (), as published by the Mexican Stock Exchange on a daily basis.
To test this work’s proposal, three portfolios that represent the performance that savers’ portfolios could have had from January 2010 to December 2014, had they invested their resources under the following three sets of conditions (scenarios), are simulated:
1. An “uninformed” scenario, where investment levels respond to the net asset value of each SIEFORE observed at the end of the previous month, following Calderón-Colín et al. (2009).
2. A “partially informed” scenario, where savers have access to a set of information similar to that presented in Table 4, the assumption being that, while savers have access to performance data, and are aware of the prevailing market conditions, they face an imperfect information flow and suffer from other externalities, such as: a) the fact that their SIEFORE is managed by a financial institution that takes advantage of back-to-back activities, including other financial products such as loans or insurance (i.e., the saver is tied to that SIEFORE due to associated contracts); b) savers can only change their SIEFORE investments once a year, due to legal restrictions; c) they are influenced by intensive marketing efforts (as suggested by Roberto Calderón-Colín et al. (2009). Nowadays, Mexican law allows Mexican pension savers to transfer their savings from one SIEFORE to another only once a year. While this measure aims to protect savers, avoiding the risk of a potential market impact on their savings, and to minimize potential irregular marketing actions, the legal limitation represents an externality that impedes the flow of resources to the best performing SIEFOREs. This scenario is simulated with the algorithm described in Appendix A.
3. A “completely informed scenario” where the legal restriction of transferring savings from one SIEFORE to another only once a year is replaced with the possibility to change them once a month, so all savers act rationally and change their savings to the best performing SIEFORE during “fair performance” and “poor performance” periods, based on information similar to that in Table 4. This scenario is modeled with the decision-making algorithm presented in Appendix B.
The simulated “all savers” portfolio accumulated returns, for each of the three scenarios, and for the whole period, is presented in Table 5:
Table 5
Performance of Simulated Portfolios for Each SIEFORE Type Under Different Scenarios
(Accumulated Returns from Nov. 30 2008 through Dec. 31 2014)
SB1 |
SB2 |
SB3 |
SB4 |
|
1. Simulated Uninformed Decisions |
35.9223 |
49.1748 |
48.7185 |
54.8667 |
2. Simulated Informed Decisions |
49.3851 |
51.7513 |
51.7333 |
51.0524 |
3. Simulated Fully Informed Decisions |
40.3401 |
106.7983 |
106.7983 |
149.4667 |
Difference between 1. and 2. |
13.4628 |
2.5765 |
3.0148 |
-3.8143 |
Difference between 1. and 3. |
4.4178 |
57.6235 |
58.0798 |
94.6000 |
Difference between 2. and 3. |
-9.0450 |
55.0470 |
55.0650 |
98.4143 |
Source: Authors’ own simulations, according to the definition of the theoretical scenarios.
The difference in returns observed among the three scenarios is very significant, especially between the uninformed and the fully informed scenarios (1. and 3.) and the informed and fully informed scenarios (2. and 3.). These results are in line with the findings of Calderón-Colín et al. (2009) and show the huge opportunity costs of the uninformed decision-making process.
Competition among pension fund managers should improve the pension-savings portfolios’ performance in Mexico. However, as Calderón-Colín et al. (2009) and Jorge Guillén (2011) point out, there is little competition among Mexican pension funds (SIEFOREs) due to informational asymmetry and to the absence of legal incentives that enhance it.
Our results provide guidelines for future public policy and regulatory initiatives, including the recommendation that a mean-variance (risk-return) performance measurement for each SIEFORE should be published to promote informed decisions by savers, and to encourage competition among pension fund managers. We also recognize some limitations in the study, such as the short period of observations, and the choice of the Markov-Switching performance of the Max Sharpe benchmark portfolio instead of other MS multifactor models (e.g., Marcelle Chauvet, 2000). These areas of potential extension on the subject open new opportunities to explore methods to improve the performance of defined contribution pension fund portfolios in countries that have adopted this system.
Albo, Adolfo, Fernando González, Ociel Hernández, Carlos A. Herrera, & Ángel Muñoz. (2007). Hacia el Fortalecimiento de los Sistemas de Pensiones en México. BBVA- Research. Mexico: BBVA. Retrieved from: http://www.bbvaresearch.com/
Alexander, Carol, & Anca Dimitriu. (2005). Indexing, Cointegration and Equity Market Regimes. International Journal of Finance & Economics, 10 (3), 213–231. https://doi.org/10.1002/ijfe.261
Alonso, Javier, Carmen Hoyo, & David Tuesta. (2015). A Model for the Pension System in Mexico: Diagnosis and Recommendations. Journal of Pension Economics and Finance, 14(1), 76–112. http://doi.org/10.1017/S147474721400016X
Attia, Nicole & Valérie Berenger. (2007). Social Protection Convergence in the European Union: Impact of Maastricht Treaty. Panoeconomicus, 54(4), 469–486. http://dx.doi.org/10.2298/PAN0704469A
Bailey, Jeffery V. (1992). Evaluating Benchmark Quality. Financial Analysts Journal, 48(3), 33-39. http://dx.doi.org/10.2469/faj.v48.n3.33
Berstein, Solange & Ruiz. (2005). Sensibilidad de la Demanda con Consumidores Desinformados: El Caso de las AFP en Chile. Serie Documentos de Trabajo, No. 4, Superintendencia de Pensiones, Santiago de Chile.
Blommestein, H. J. (1997). Institutional Investors, Pension Reform and Emerging Securities Markets. In The Development of Securities Markets in Emerging Markets: Obstacles and Preconditions for Success: 1–52. Paris, France.
Brandt, Michael W & Qiang Kang (2004). On the Relationship Between the Conditional Mean and Volatility of Stock Returns: A Latent VAR Approach. Journal of Financial Economics, 72(2), 217–257. http://dx.doi.org/10.1016/j.jfineco.2002.06.001
Brooks, Chris & Gita Persand. (2001). The Trading Profitability of Forecasts of the Gilt–Equity Yield Ratio. International Journal of Forecasting, 17(1), 11–29.
Calderón-Colín, Roberto, Enrique Domínguez & Moisés Schwartz (2010). Consumer Confusion: The Choice of Pension Fund Manager in Mexico. Journal of Pension Economics and Finance, 9(1), 43–74. https://doi.org/10.1017/S1474747209004004
Chauvet, Marcelle (2000). An Econometric Characterization of Business Cycle Dynamics with Factor Structure and Regime Switching. International Economic Review, 10(2), 127–142. http://dx.doi.org/10.2307/2527348
CONSAR (2014). SIEFOREs Historical Prices. https://www.consar.gob.mx/
CONSAR (2016). SIEFOREs Historical Prices. https://www.consar.gob.mx/
Cosslett, Stephen R. & Lung-Fei Lee (1985). Serial Correlation in Latent Discrete Variable Models. Journal of Econometrics, 27, 79-97. http://dx.doi.org/10.1016/0304-4076(85)90045-4
De la Torre, Óscar V., Evaristo Galeana-Figueroa, María Isabel Martínez-Torre Enciso & Dora Aguilasocho-Montoya (2015). A Minimum Variance Benchmark to Measure the Performance of Pension Funds in Mexico. Contaduría y Administración UNAM, 61(3), 593-614. http://dx.doi.org/10.1016/j.cya.2015.05.009
Fuentes, Eduardo, Alicia García-Herrero & José Escrivá (2010). Las Reformas de los Sistemas de Pensiones en Latinoamérica. Retrieved from: http://www.bbvaresearch.com
Goldfeld, Stephen M. & Richard Quandt 1973. A Markov Model for Switching Regressions. Journal of Econometrics, 1, 3-16. http://dx.doi.org/10.1016/0304-4076(73)90002-X
Guillén, Jorge (2011). Latin American Private Pension Funds’ Vulnerabilities. Economía Mexicana. Nueva Época, XX (2), 357–378.
Hamilton, James D. (1989). A New Approach to the Economic Analysis of Non-stationary Time Series and the Business Cycle. Econometrica, 57(2), 357–384. http://dx.doi.org/10.2307/1912559
Hamilton, J. D. (1994). Time Series Analysis. Princeton: Princeton university press.
Hauptmann, Johannes, Anja Hoppenkamps, Aleksey Min, Franz Ramsauer, & Rudi Zagst (2014). Forecasting Market Turbulence Using Regime-Switching Models. Financial Markets and Portfolio Management, 28(2), 139–164. http://dx.doi.org/10.1007/s11408-014-0226-0
James, Estelle (2005). Reforming Social Security: Lessons From 30 Countries (No. 277). NCPA Policy Reports (Vol. 75251). Dallas, TX, U.S.A. www.ncpa.org/pub/st/st277
Lettau, Martin & Sydney Ludvigson (2010). Measuring and Modeling Variation in the Risk-Return Trade-Off. In Aït-Sahalia, Y. and Peter Hansen, L. (eds.) Handbook of Financial Econometrics; Tools and Techniques, vol. 1, North-Holland, Amsterdam.
Ludvigson, Sydney & Serena Ng. (2007). The Empirical Risk-Return Relation: a Factor Analysis Approach. Journal of Financial Economics 83(1), 171 – 222. http://dx.doi.org/10.1016/j.jfineco.2005.12.002
Lustig, Hanno N. & Adrien Verdelhan (2012). Business Cycle Variation in the Risk-Return Trade-Off. Journal of Monetary Economics, 59(Supplement), 535-549. http://dx.doi.org/10.1016/j.jmoneco.2012.11.003
Martínez, Marissa & Francisco Venegas-Martínez (2014). Análisis del Riesgo de Mercado de los Fondos de Pensión en México. Un Enfoque con Modelos Autorregresivos. Contaduría y Administración, 59(3), 165–195. https://doi.org/10.1016/S0186-1042(14)71269-0
Neftci, Salih (1984). Are Economic Time Series Asymmetric Over the Business Cycle? Journal of Political Economy, 92, 307-328. http://dx.doi.org/10.1086/261226
Peinado, Patricia, and Felipe Serrano (2011). A Dynamic Analysis of the Effect of Social Security Reform on Spanish Widow Pensioners. Panoeconomicus, 58(5), 759–771. http://dx.doi.org/10.2298/PAN1105759P
Peinado, Patricia, and Felipe Serrano (2014). Fiscal Crisis, Social Security Reform and Vulnerable Population. Panoeconomicus, 61(SPEC. ISSUE), 129–143. http://dx.doi.org/10.2298/PAN1401129P
Santillán-Salgado, Roberto J., David López & Justo Montenegro (2010). Las Administradoras de Fondos de Pensiones y el Desarrollo del Mercado de Capitales en Chile. Ensayos Revista de Economía, Vol. XXIX, No. 2, 53-76. RePEc: ere:journl:v:xxix:y:2010:i:2:p:53-76
Santillán-Salgado, Roberto J., Marissa Martínez-Preece & Francisco López-Herrera (2016). Análisis Econométrico del Riesgo y Rendimiento de las SIEFORES. Revista Mexicana de Economía Y Finanzas, 11(1), 29–54. http://dx.doi.org/10.21919/remef.v11i1.76
Schmidt-Hebbel, Klaus (1999). Does Pension Reform Really Spur Productivity, Saving, and Growth?, Documentos de Trabajo, No. 33, Banco Central de Chile.
Semler, Willi (2011). Asset Prices, Booms and Recessions. Third Edition, Springer-Verlag, Heidelberg, Berlin.
Tang, Yi & Robert F. Whitelaw (2011). Time-varying Sharpe Ratios and Market Timing. Quarterly Journal of Finance, 1 (3), 465-493. https://doi.org/10.1142/S2010139211000122
Turner, John A, & Gerard Hughes (2008). Large Declines in Defined Benefit Plans Are Not Inevitable: The Experience of Canada, Ireland, the United Kingdom, and the United States. Pensions Institute Discussion Papers, (No. PI-0821). Retrieved from: http://www.pensions-institute.org/workingpapers/wp0821.pdf
Williamson, John B., Meghan Price, & Ce Shen (2012). Pension Policy in China, Singapore, and South Korea: An Assessment of the Potential Value of the Notional Defined Contribution Model. Journal of Aging Studies, 26(1), 79–89. http://dx.doi.org/10.1016/j.jaging.2011.08.002
Appendix A. Using Markov-Switching Sharpe Ratios in Partially Informed Scenarios

1. Full-time Professor and Researcher at the Accounting and Management Sciences, Faculty of Michoacán State University, Morelia (Mexico). E-mail: oscar.delatorre.torres@gmail.com
2. PhD in Direction and Planning of Tourism (University of Vigo, Spain). Associate Professor and Researcher at the Department of Financial Economics and Accounting. Faculty of Business, Finance and Tourism, University of Extremadura, Cáceres (Spain). Email: pepealvarez@unex.es . Corresponding author.
3. Full-time Professor and Researcher at Finance Department, EGADE Business School, Tecnológico de Monterrey (México). E-mail: roberto.santillan@itesm.mx
4. Full-time Professor and Researcher at the Facultad de Contaduría y Administración, Universidad Nacional Autónoma de México, (México). E-mail: francisco_lopez_herrera@yahoo.com.mx